01a. PEN K12 (Canada 1969) Find all functions  such that for all
such that for all  :
: 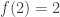 ,
, 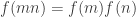 ,
,  .
.
01b. Let  be a function satisfying the conditions:
be a function satisfying the conditions:
(a) 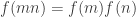 for all relatively prime
for all relatively prime  and
and  , and
, and
(b)  for all positive integers
for all positive integers  .
.
Show that there is a constant 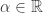 such that
such that  for all
for all  .
.
Here is the official solution: PEN01S
An alternative solution is here: CMO 2003 (problem 4)
You can also disscuss the problems here!
References
[1] P. Erdos, On the distribution function of additive functions, Ann. of Math., 47(1946), 1-20
[2] E. Howe, A new proof of Erdos’s theorem on monotone multiplicative functions, Amer. Math. Monthly, 93(1986), 593-595
[3] L. Moser and J. Lambek, On monotone multiplicative functions, Proc. Amer. Math. Soc., 4(1953), 544-545
Acknowledgement. We would like to express our gratitude to Andrei Frimu who helped us preparing the solutions and Orlando Doehring who offered me the solution file of CMO 2003.
Read Full Post »
and
satisfying
.